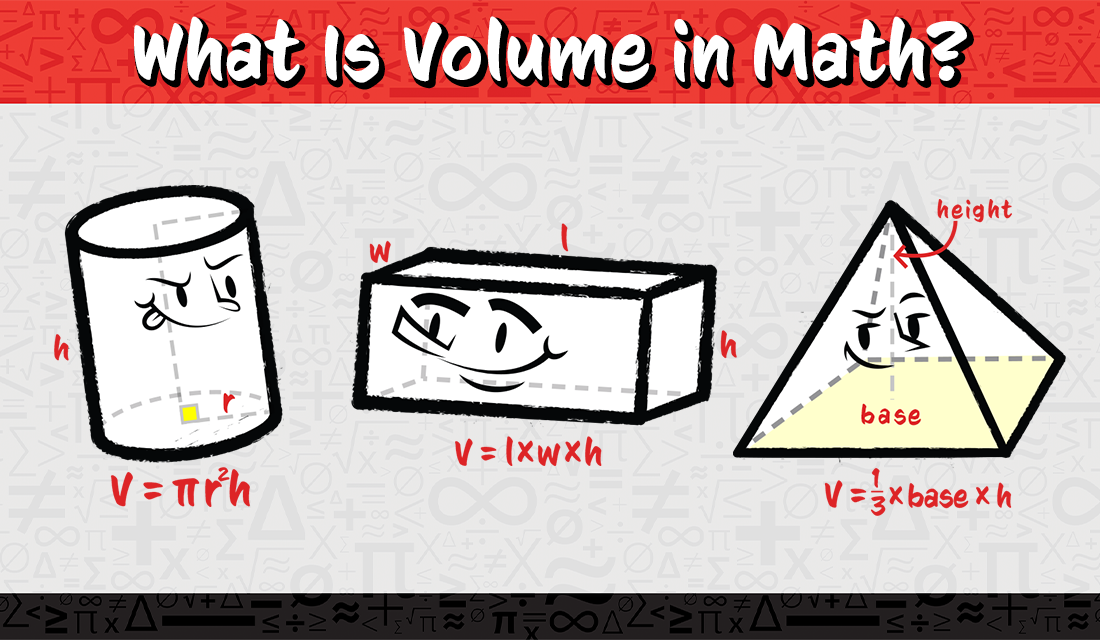
From the glass on your table to the skyscrapers that reach for the clouds, volume helps us understand and measure the scale of our physical world.
We’ve put together a comprehensive guide to everything you need to know to get started with volumes in math.
Read on to find simple definitions and explanations, learn to calculate volumes of 3D objects, see solved examples, and test your knowledge with bonus practice exercises.
What Is Volume?
Volume is the measure of how much space an object takes up in three dimensions. Sometimes, people also use the term "capacity" to talk about volume, especially when discussing how much a container like a cup or a box can hold.
To put it differently, if you understand volume, you can figure out how many candies fit in a jar, how many books fill a shelf, or how much water a swimming pool can contain.

Knowing how to calculate volume can help us figure out about how many jellybeans fit into a jar.
In this short video, one of the founders of Mathnasium Larry Martinek explains volume using Rubik's® Cubes.
What Are the Volume Units?
Just like we use inches, feet, and miles to measure length, and ounces and pounds to measure weight, volume also has its own set of measuring units.
When measuring the volume of three-dimensional (3D) objects, we use cubic units.
- In the customary system, we use cubic inches (in³), cubic feet (ft³), cubic yards (yd³), and cubic miles (mi³) to measure volume.
- Similarly, in the metric system, we use cubic centimeters (cm³), cubic meters (m³), and cubic kilometers (km³).
As you might have guessed, the reason why we use cubic units to measure volume is because we are measuring three-dimensional spaces. When we are measuring the area of two-dimensional (2D) spaces like squares or rectangles, for example, we use square units such as ft2 or m2.
Find Top-Rated Geometry Tutors at Mathnasium
How to Find the Volume of Cubes, Prisms & Cylinders
As 3D objects can vary greatly in shape, there is no universal formula for calculating their volume, but there is a universal logic we can follow to calculate the volume of cubes, prisms, and cylinders.
To find the volume of any of these 3 shapes, all we have to do is calculate the area of the base and multiply it by the height.
Let's put this into action:
Finding Volumes of Cubes
Cubes are special types of rectangular prisms where all sides are equal in length. You can find cubes in everyday items like dice, building blocks, and sugar cubes!
To find the volume of a cube, we should calculate the area of its base and multiply it by the height.
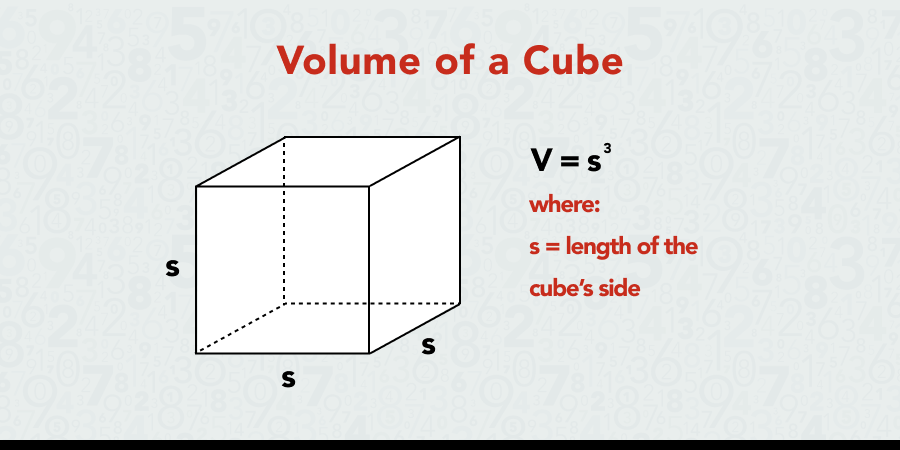
Let’s say the length of the side is 7 inches.
The area of a square base is 7 x 7, or 72.
As all the sides are equal in length, the height of the cube is also 7
.
So to calculate the volume of the square, we should multiply
by 72 by 7
, which we can also express as 73
. The volume of our square is 343 cubic inches (in3).
The formula we use to calculate the volume (V
) of a cube is simply the length of its side cubed (s3
):
V = s3
For example, let's consider a cube with side length: s = 4 in
To find its volume, we use this formula: V = s3
V = 43 or V = 4 x 4 x 4
V = 64 in3
The volume of our cube in this case is 64 in³.
Finding Volumes of Rectangular Prisms

A rectangular prism is a three-dimensional shape with six faces, all of which are rectangles. You can see rectangular prisms all around, like in cardboard boxes, shoeboxes, and bricks!
Rectangular prisms have three pairs of parallel faces:
- Top and bottom
- Front and back
- Left and right side
The faces in each pair are the same shape and size, so they have the same area.
To calculate the volume of a rectangular prism, we start by defining its length (l)
, height (h)
, and width (w)
.
To find the base, we multiply the length by the width l x w.
To find volume, we multiply the base l x w
by the height: l x w x h
And we found the formula for calculating the volume of prisms:
V = l x w x h
Let's try this example.
- The length of our rectangular prism is 6 inches (l = 6 in)
- The width is 3 inches (w = 3 in)
- The height is 2 inches (h = 2 in)
To calculate the volume of the prism, we multiply all three values:
V = 6 x 3 x 2
V = 36 in3
Finding Volumes of Cylinders
A cylinder is a three-dimensional shape with two circular bases of the same size that are parallel to each other and connected by a curved surface. For example, you can see cylinders in objects like paper towel tubes or soda cans.
To calculate the volume of a cylinder, we need to know the radius of its base and its height.
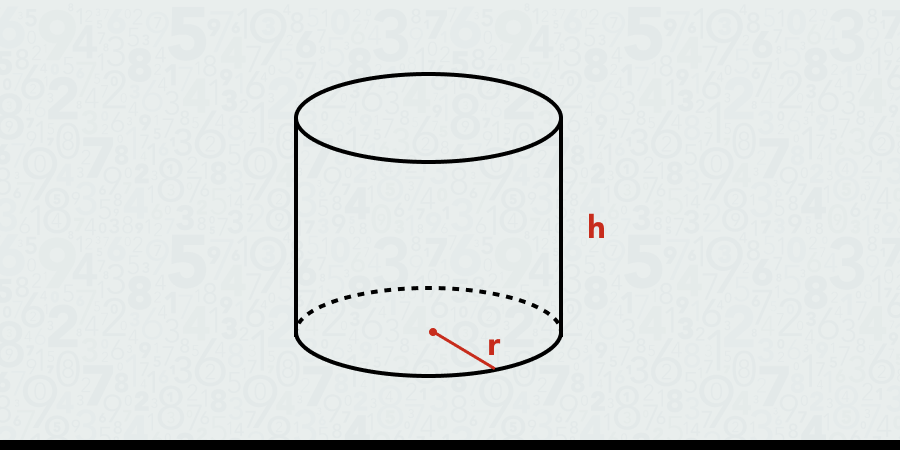
To find the formula for the volume of a cylinder, let’s start by calculating the area of its base.
As the base of a cylinder is a circle, to calculate the area we multiply π by the squared radius (r2): π x r2.
Now all we have to do to find the volume is to multiply the area of the base with the height of the cylinder: π x r2 x h
There we have it! The formula for calculating the volume of a cylinder is:
V = π x r2 x h
Let’s put it into action!
If you remember your lessons on the area of the circle, you will recall the Greek letter π, or “Pi”, and that its value is approximately 3.14.
In our examples, we will leave the volume in terms of π (pi) to simplify calculations.
What we are missing now is to define the radius (r) of the base and the height of our cylinder (h), so let’s say they measure:
Radius r = 3 in
Height h = 5 in
To calculate its volume, we use the formula from earlier:
V = π x r2 x h
Substitute the values:
V = π x 32 x 5
V = π x 9 x 5
V = π x 45
V = 45π
So, the volume of our cylinder is 45π in³.
How to Find the Volume of Pyramids, Cones & Spheres
Ready for the next challenge?
Let’s level up your geometry skills and tackle the volumes of pyramids, cones, and spheres.
Finding Volumes of Pyramids
A pyramid is a three-dimensional shape with a flat base and triangular faces that meet at a single point called the apex.
The base of a pyramid is the flat surface that the pyramid sits on. The surface can vary in shape, from triangles to squares, and beyond.
The height of a pyramid is the vertical distance from its apex (top) down to its base, measured along a straight line perpendicular to the base.

To find the volume of a pyramid, just like with prisms and cylinders, we need to know the area of its base (
) and its height.
But, unlike prisms and cylinders, pyramids do not have two parallel bases, so our volume formula will differ slightly.
We will multiply the base and the height of the pyramid by 1/3
, like so:
V = 1/3 x B x h
For example, let's say our pyramid has a square base with these dimensions:
- Base side length: s = 3 in
- Height: h = 6 in
To find its volume, we use the formula: V = 1/3 x B x h
For a square base, the base area is calculated as: B = s2
So, we get
V = 1/3 x 33 x 6
V = 1/3 x 54
V = 18 in3
So, the volume of our square-based pyramid is 18 in³.
2. Finding Volumes of Cones
A cone is a 3D shape with a circular base and a curved side that gets smaller as it goes to a point at the top, called the apex.
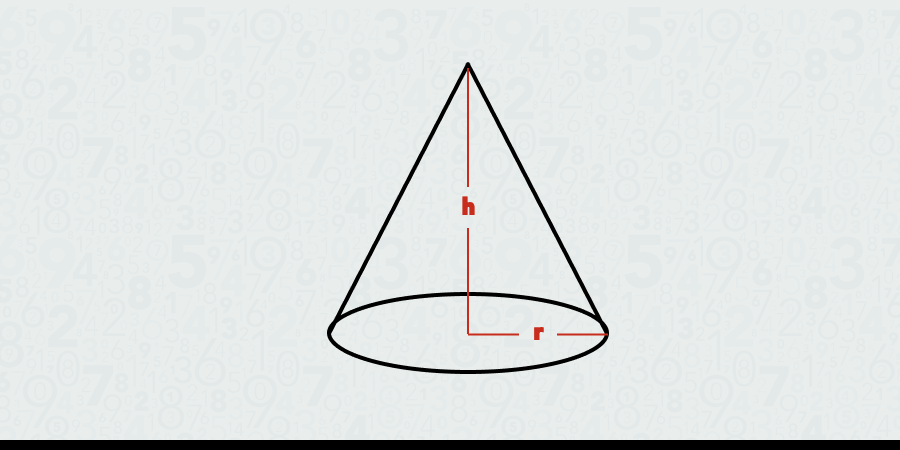
To find the volume of a cone, we need to know the radius (r)
of its circular base and its height (h). Then, we find the area of the base, multiply it by the height of the cone and by 1/3
.
V = 1/3 x π x r2 x h
You may have noticed that this is the same formula as the pyramid (base x height), except in this case the base is a circle, so we will calculate the area using: π x r2
Let's try our hands at this example.
If we know the radius
is 4 in, and the height(h)
is 6 in, we can find the volume of the cone.
When we substitute the formula above with the given values, we get:
V = 1/3 x π x r2 x h
V = 1/3 x π x 16 x 6
V = 1/3 x π x 96
V = 96/3 x π
V = 32π
The volume of our cone is 32π in³.
3. Finding Volumes of Sphere
A sphere is a perfectly round 3D shape where every point on the surface is the same distance from the center.
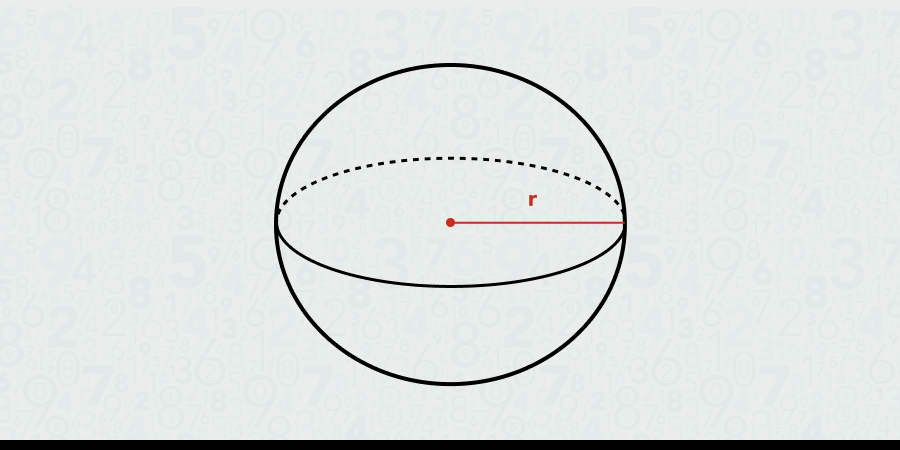
To calculate the volume of a sphere, we need to know its radius (r)
. The formula for the volume of a sphere is V = 4/3 x π x r3.
Let’s use an example to illustrate. If a sphere has a radius of 3 in, we can find its volume using the formula above.
V = 4/3 x π x r3
V = 4/3 x π x 27.
V = 4 x27/3 x π
V = 108/3 x π
V = 36 x π
V = 36π
Finally, we get the volume of our sphere and it’s 36π in³.
Solved Examples of Volume Exercises
Let's explore some solved examples to reinforce our knowledge.
Example 1: Cube
Most of our students love cubes because their volumes are one of the easiest to find!
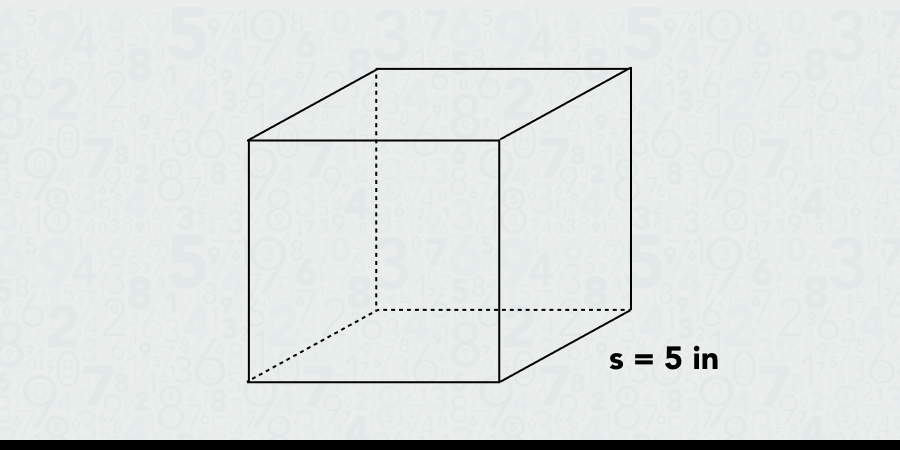
Why are they easy to find?
Because all of their sides are the same length, so if we know one, we know them all.
In this example, the side of our cube measures at 5 in.
V = s3
Substituting the values, we get:
V = 53
V = 5 x 5 x 5
V = 125 in3
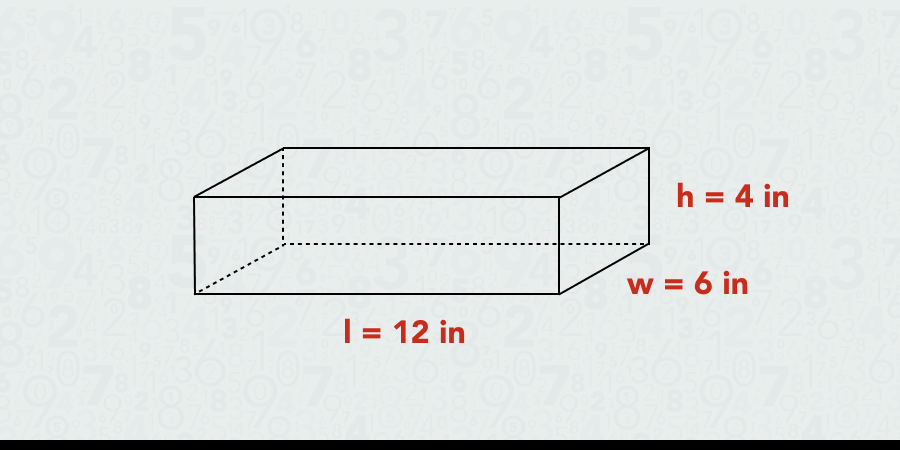
Example 2: Rectangular Prism
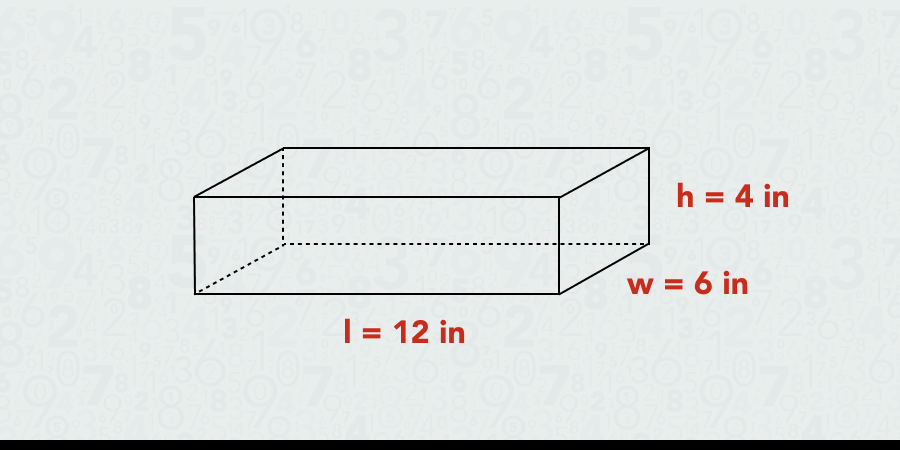
Based on the information you see in the image, how would you calculate the volume of this rectangular prism?
Let’s start by taking note of the dimensions:
- Length (l) = 12 in
- Width (w) = 6 in
- Height (h) = 4 in
We remember the rectangular prism volume formula:
V = l x w x h
When we insert the values into our formula, we get:
V = 12 x 6 x 4
V = 288 in3
Example 3: Cylinder
Cylinders might look scary because of their formula, specifically the π, but once you get used to the formula, their volumes are pretty easy to calculate.
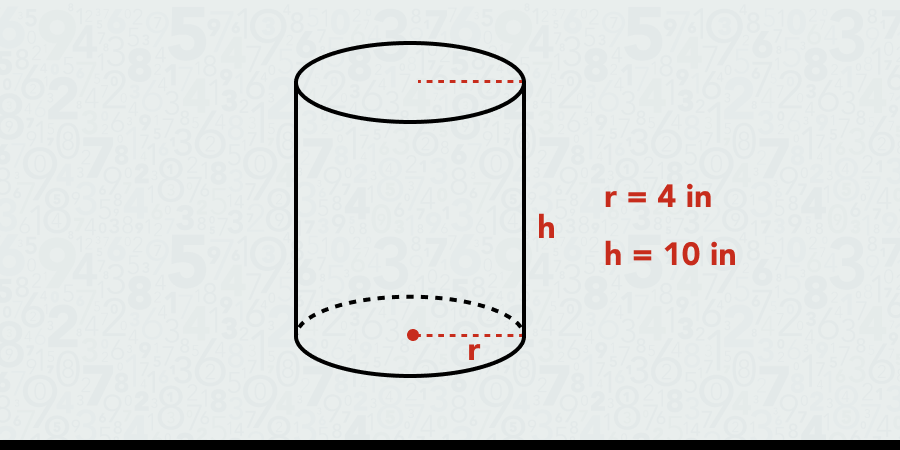
In this case, our cylinder has:
- Radius: 4 inches
- Height: 10 inches.
The formula for measuring the volume of a cylinder is: V = π x r2 x h
Remembering that π ≈ 3.14 and substituting the given values, we get:
V = π x 42 x 10
V = π x 16 x 10
V = π x 160
V = 160π in3
Example 4: Pyramid with a Rectangular Base
In this example, we have a pyramid with a rectangular base.
We know the universal formula for pyramid volume is V = 1/3 x B x h
where B represents the area of the base.
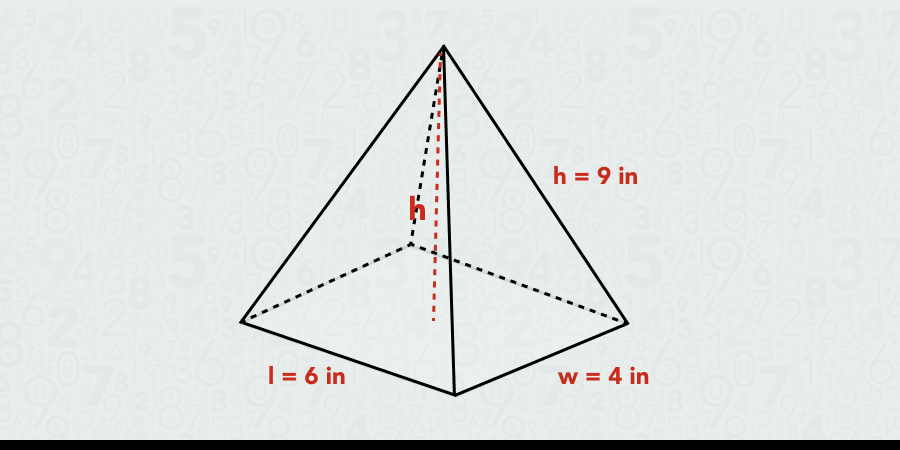
Let’s take note of the dimensions:
- Height: 9 in
- Length: 6 in
- Width: 4 in
Since we’re dealing with a rectangular base, we will get the value of the base (B) by multiplying the length and width of the rectangle, so our formula will look like this:
V = 1/3 x B x h
V = 1/3 x (l x w) x h
Now let’s insert our values and calculate the volume of our pyramid:
V = 1/3 x 6 x 4 x 9
V = 1/3 x 216
V = 72 in3
Example 5: Cone
Now, let's work out the volume of this cone together.
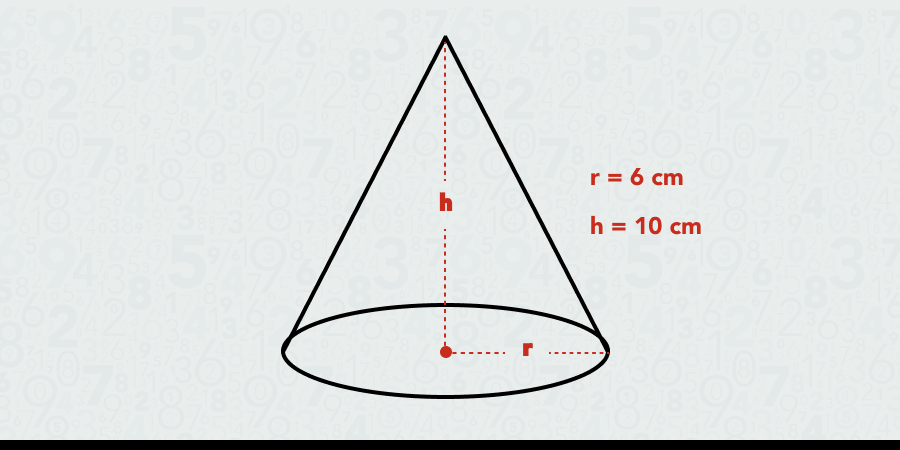
For cone volume, we use the formula: V = 1/3 x π x r2 x h
Write down the dimensions:
- Radius (r): 6 in
- Height (h): 10 in
Let’s substitute the values:
V = 1/3 x π x 36 x 10
V = 1/3 x π x 360
V = 360/3 x π
V = 120 x π
V = 120π in3
Example 6: Sphere
Time to tackle spheres—those perfectly round wonders of geometry!
To get the volume of a sphere, we follow the formula: V = 4/3 x π x r3

Since our radius is 6 in, we can substitute the value right away:
V = 4/3 x π x 63
V = 4/3 x π x 216
V = 4 x 216/3 x π
V = 864/3 x π
V = 288 x π
V = 288π3 in3
Quiz – Test Your Knowledge of Volume
Create your own user feedback survey
Frequently Asked Questions About Volume
Here are the questions we often get from our students when learning about volumes in math:
1. How is volume different from area?
Volume is the space inside a three-dimensional object, like a box, while area measures the space covering a two-dimensional surface, like the floor of a room.
To measure volume, we use cubic units (in³, ft³, etc.) and to measure area we use square units (in², ft², etc.).
2. Can the volume change?
Yes, volume can change! For example, when you pour water into a cup, the volume in the cup increases because it’s holding more liquid.
Similarly, if you squeeze a balloon, its volume decreases because you're reducing the amount of air inside.
3. How is volume used in everyday life?
Perhaps one of the best examples of how we use volumes in everyday life is cooking.
We use volume to measure ingredients like flour, sugar, and liquids.
When a recipe says "1 cup of flour" or "1/2 cup of milk," it's talking about how much space those ingredients take up in a measuring cup.
These precise measurements allow us to replicate the dishes we love.
We also use volumes to measure how much:
- Water we’ll need to fill a pool
- Fuel we can put into our car
- Space we can occupy in a storage unit and so on
Learn & Master Volume with Top-Rated Geometry Tutors Near You
Mathnasium’s specially trained geometry tutors work with K-12 students regardless of their skill level.
Explore our approach to elementary school tutoring:
Our tutors assess each student’s current skills and considers their unique academic goals to create personalized learning plans that will put them on the best path towards math mastery.
Whether you are looking to catch up, keep up, or get ahead in your math class, find a Mathnasium Learning Center near you, schedule an assessment, and enroll today!
Find a Math Tutor Near You