See Our Method 
OUR RESULTS
The results are transformative - families will see measurable changes in attitude, confidence, and school progress.
See Our Results
Get Started by Finding a Local Centre
For more than a decade, the Mathnasium Method™ has transformed the way kids understand and appreciate math. Larry Martinek, creator of the Mathnasium Method, has spent 40+ years designing, developing, and refining this approach based on his extensive experience teaching math to kids. We build math knowledge upon what they already know—this helps kids learn and starts boosting confidence right away.
We take our students on a journey of learning – through assessment, customized learning paths and targeted lessons for understanding and comprehension.
1
We begin with a comprehensive assessment, which includes both a verbal and written component, to pinpoint their exact strengths and weaknesses.
2
This plan is created for each child based on their assessment, so they will truly learn and grow in their mathematical thinking.
3
Our expert instructors don’t just teach student to memorize or calculate; they teach them to truly understand the way math works.
4
As students achieve their goals, they are reassessed and move on to new challenges.
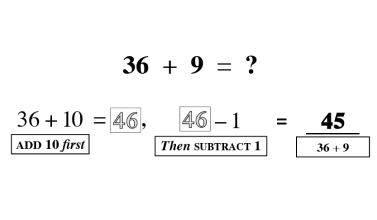
This is the key to success in math—the understanding of what numbers mean and how they work together. And Number Sense isn't just for young kids. We work on these topics through the levels shown below before moving on to other higher math disciplines.
Counting
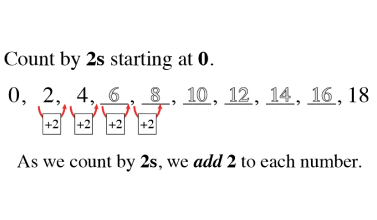
Counting is the key to unlocking addition and subtraction in early math development. At Mathnasium, our initial goal is to have a student become comfortable with counting to any number, from any number, by any number, forward and backward.
Wholes And Parts
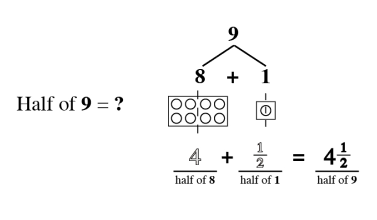
As students begin to understand the relationship between a whole and the parts, a world of mathematical concepts and exercises can be explored. Once students have mastered these skills, they have little trouble with algebraic problem-solving.
Quantity and Denomination
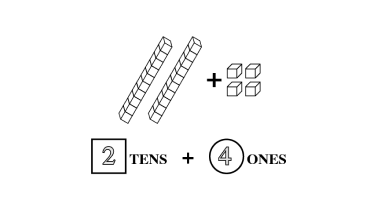
The quantity and denomination construct examine two aspects of numerical value. Quantity asks “how many” and denomination asks “of what.”
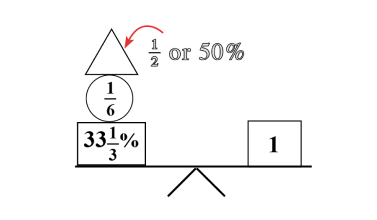
Proportional Thinking
Proportional thinking establishes a fundamental base that leads to a stronger understanding of critical concepts like ratios, direct and indirect variation, and algebraic reasoning.
The Law of SAMEness
The Law of SAMEness is a concept students naturally apply in their reasoning without being aware of it. For example, quantities of apples and bananas cannot be added together unless first being changed so that they have the same name, which is fruit.
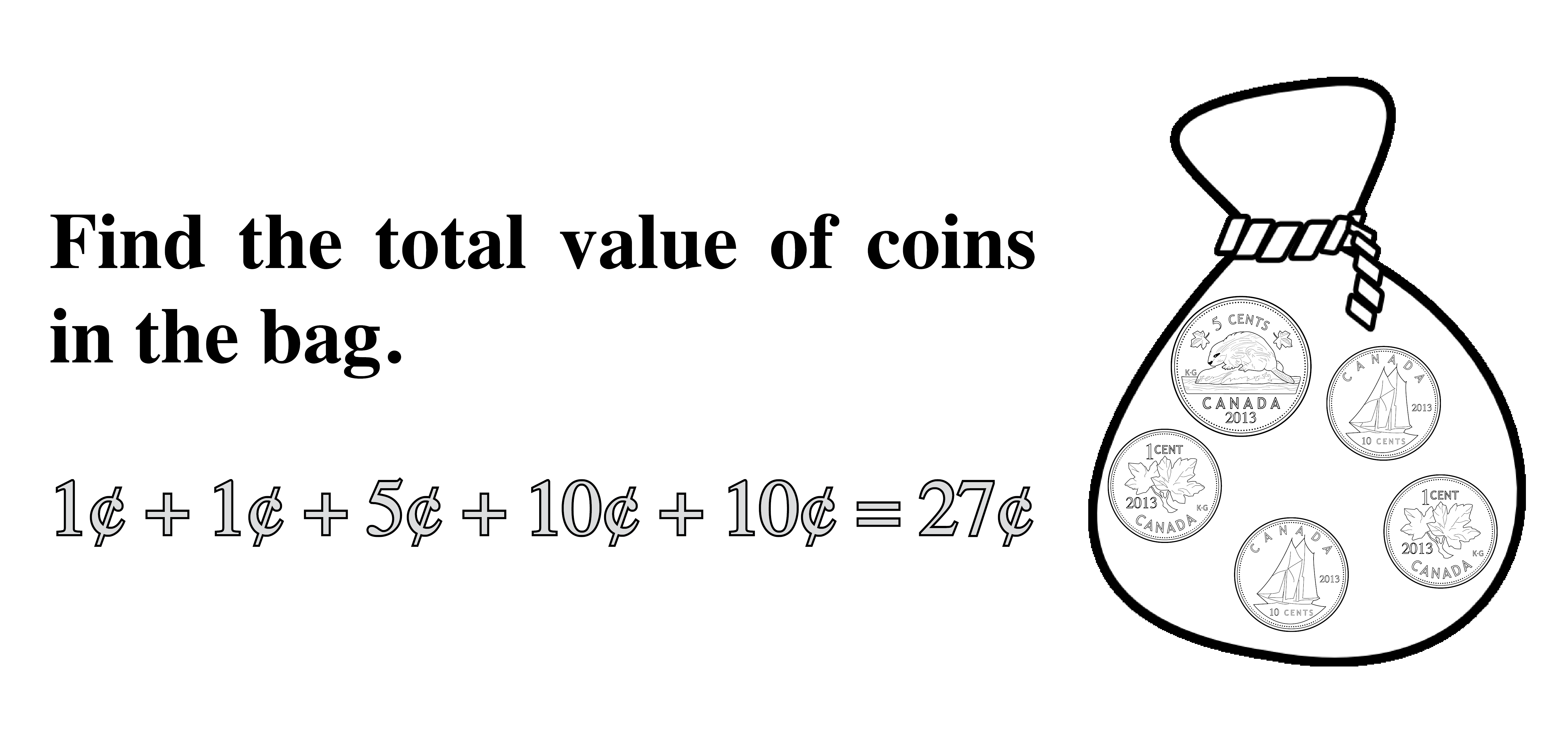
Counting
Counting is the key to unlocking addition and subtraction in early math development. At Mathnasium, our initial goal is to have a student become comfortable with counting to any number, from any number, by any number, forward and backward.
Wholes and Parts
As students begin to understand the relationship between a whole and the parts, a world of mathematical concepts and exercises can be explored. Once students have mastered these skills, they have little trouble with algebraic problem-solving.
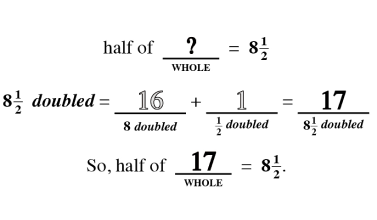
Quantity and Denomination
The quantity and denomination construct examine two aspects of numerical value. Quantity asks “how many” and denomination asks “of what.”
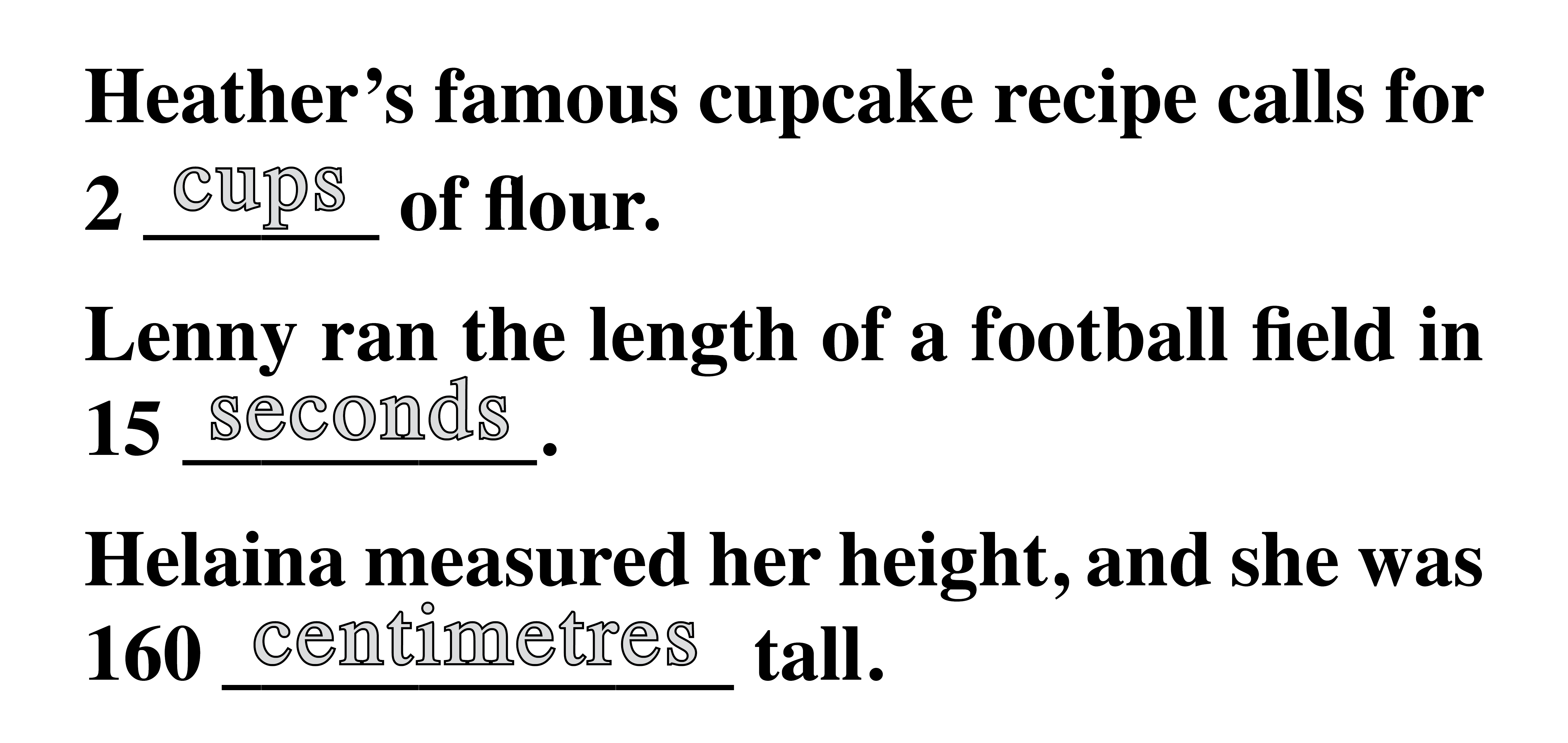
Proportional Thinking
Proportional thinking establishes a fundamental base that leads to a stronger understanding of critical concepts like ratios, direct and indirect variation, and algebraic reasoning.
The Law of SAMEness
The Law of SAMEness is a concept students naturally apply in their reasoning without being aware of it. For example, quantities of apples and bananas cannot be added together unless first being changed so that they have the same name, which is fruit.
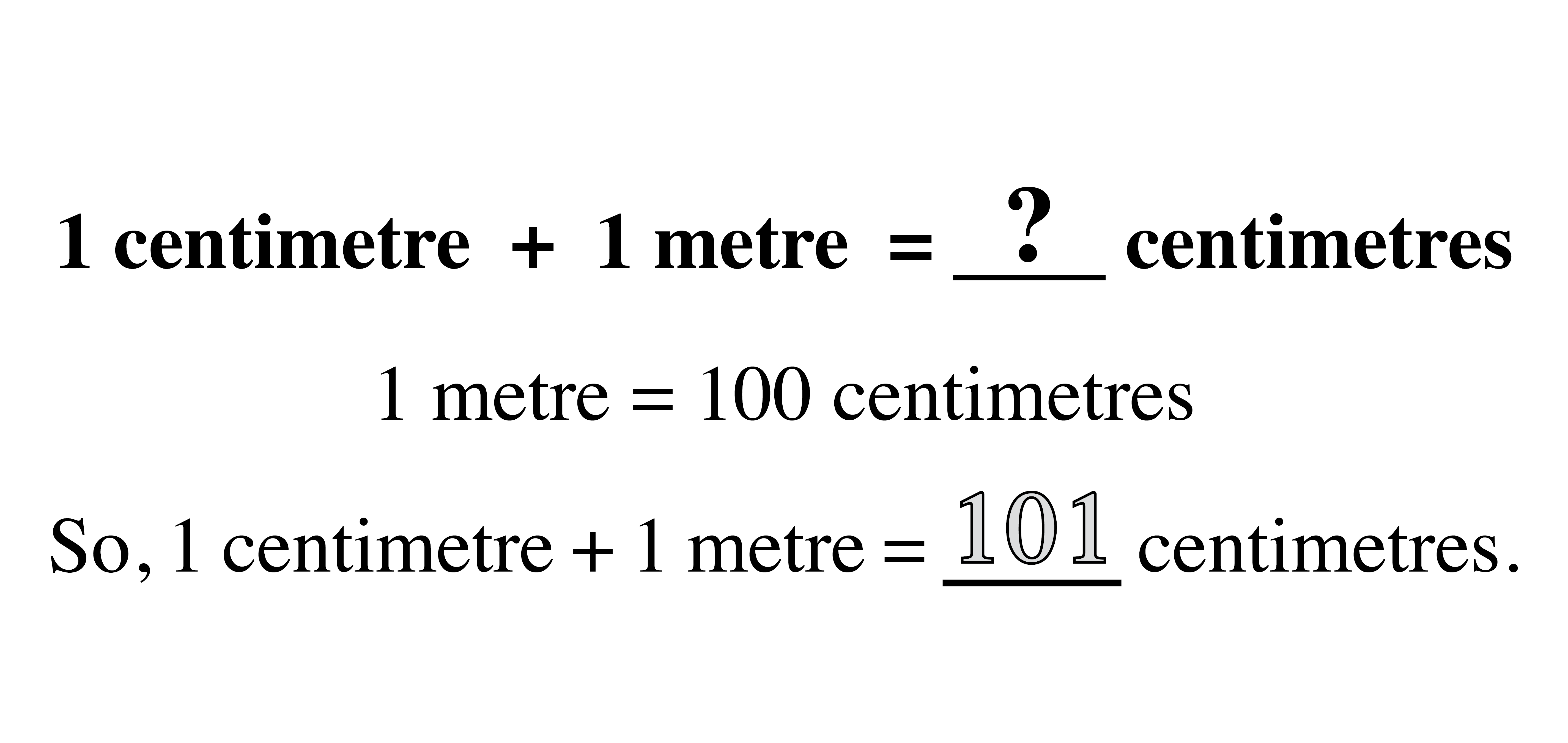
Counting
Counting is the key to unlocking addition and subtraction in early math development. At Mathnasium, our initial goal is to have a student become comfortable with counting to any number, from any number, by any number, forward and backward.
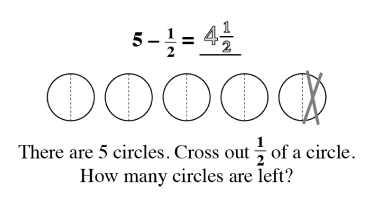
Wholes and Parts
As students begin to understand the relationship between a whole and the parts, a world of mathematical concepts and exercises can be explored. Once students have mastered these skills, they have little trouble with algebraic problem-solving.
Quantity and Denomination
The quantity and denomination construct examine two aspects of numerical value. Quantity asks “how many” and denomination asks “of what.”
Proportional Thinking
Proportional thinking establishes a fundamental base that leads to a stronger understanding of critical concepts like ratios, direct and indirect variation, and algebraic reasoning.
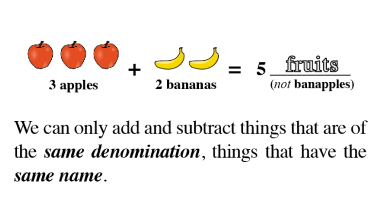
The Law of SAMEness
The Law of SAMEness is a concept students naturally apply in their reasoning without being aware of it. For example, quantities of apples and bananas cannot be added together unless first being changed so that they have the same name, which is fruit.
Counting
Counting is the key to unlocking addition and subtraction in early math development. At Mathnasium, our initial goal is to have a student become comfortable with counting to any number, from any number, by any number, forward and backward.
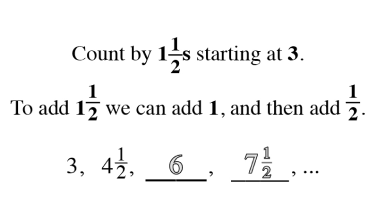
Wholes and Parts
As students begin to understand the relationship between a whole and the parts, a world of mathematical concepts and exercises can be explored. Once students have mastered these skills, they have little trouble with algebraic problem-solving.
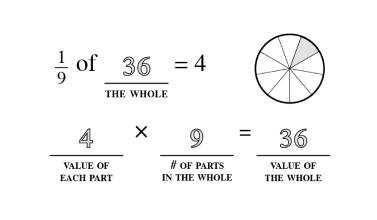
Quantity and Denomination
The quantity and denomination construct examine two aspects of numerical value. Quantity asks “how many” and denomination asks “of what.”
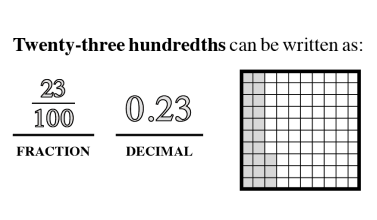
Proportional Thinking
Proportional thinking establishes a fundamental base that leads to a stronger understanding of critical concepts like ratios, direct and indirect variation, and algebraic reasoning.
The Law of SAMEness
The Law of SAMEness is a concept students naturally apply in their reasoning without being aware of it. For example, quantities of apples and bananas cannot be added together unless first being changed so that they have the same name, which is fruit.
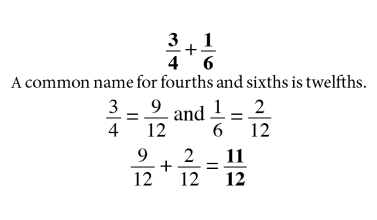
Counting
Counting is the key to unlocking addition and subtraction in early math development. At Mathnasium, our initial goal is to have a student become comfortable with counting to any number, from any number, by any number, forward and backward.
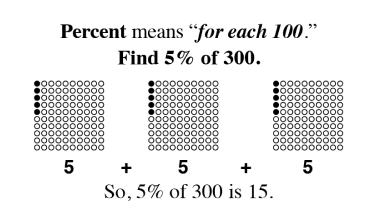
Wholes and Parts
As students begin to understand the relationship between a whole and the parts, a world of mathematical concepts and exercises can be explored. Once students have mastered these skills, they have little trouble with algebraic problem-solving.
Quantity and Denomination
The quantity and denomination construct examine two aspects of numerical value. Quantity asks “how many” and denomination asks “of what.”
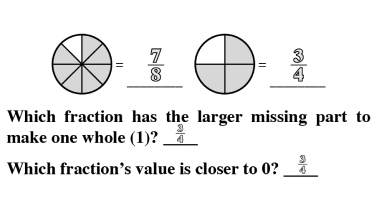
Proportional Thinking
Proportional thinking establishes a fundamental base that leads to a stronger understanding of critical concepts like ratios, direct and indirect variation, and algebraic reasoning.
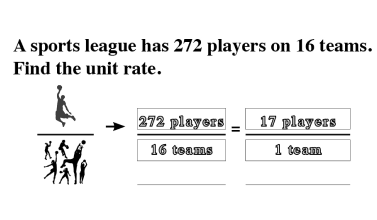
The Law of SAMEness
The Law of SAMEness is a concept students naturally apply in their reasoning without being aware of it. For example, quantities of apples and bananas cannot be added together unless first being changed so that they have the same name, which is fruit.
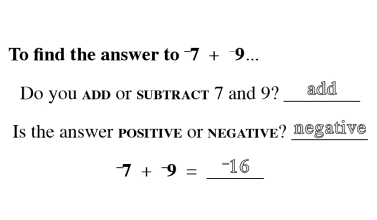
Counting
Counting is the key to unlocking addition and subtraction in early math development. At Mathnasium, our initial goal is to have a student become comfortable with counting to any number, from any number, by any number, forward and backward.
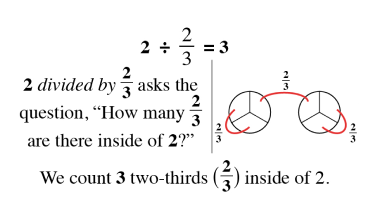
Wholes and Parts
As students begin to understand the relationship between a whole and the parts, a world of mathematical concepts and exercises can be explored. Once students have mastered these skills, they have little trouble with algebraic problem-solving.
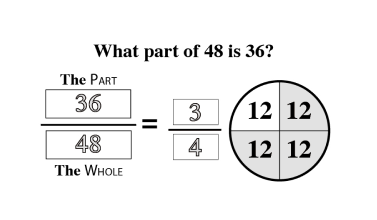
Quantity and Denomination
The quantity and denomination construct examine two aspects of numerical value. Quantity asks “how many” and denomination asks “of what.”
Proportional Thinking
Proportional thinking establishes a fundamental base that leads to a stronger understanding of critical concepts like ratios, direct and indirect variation, and algebraic reasoning.
The Law of SAMEness
The Law of SAMEness is a concept students naturally apply in their reasoning without being aware of it. For example, quantities of apples and bananas cannot be added together unless first being changed so that they have the same name, which is fruit.
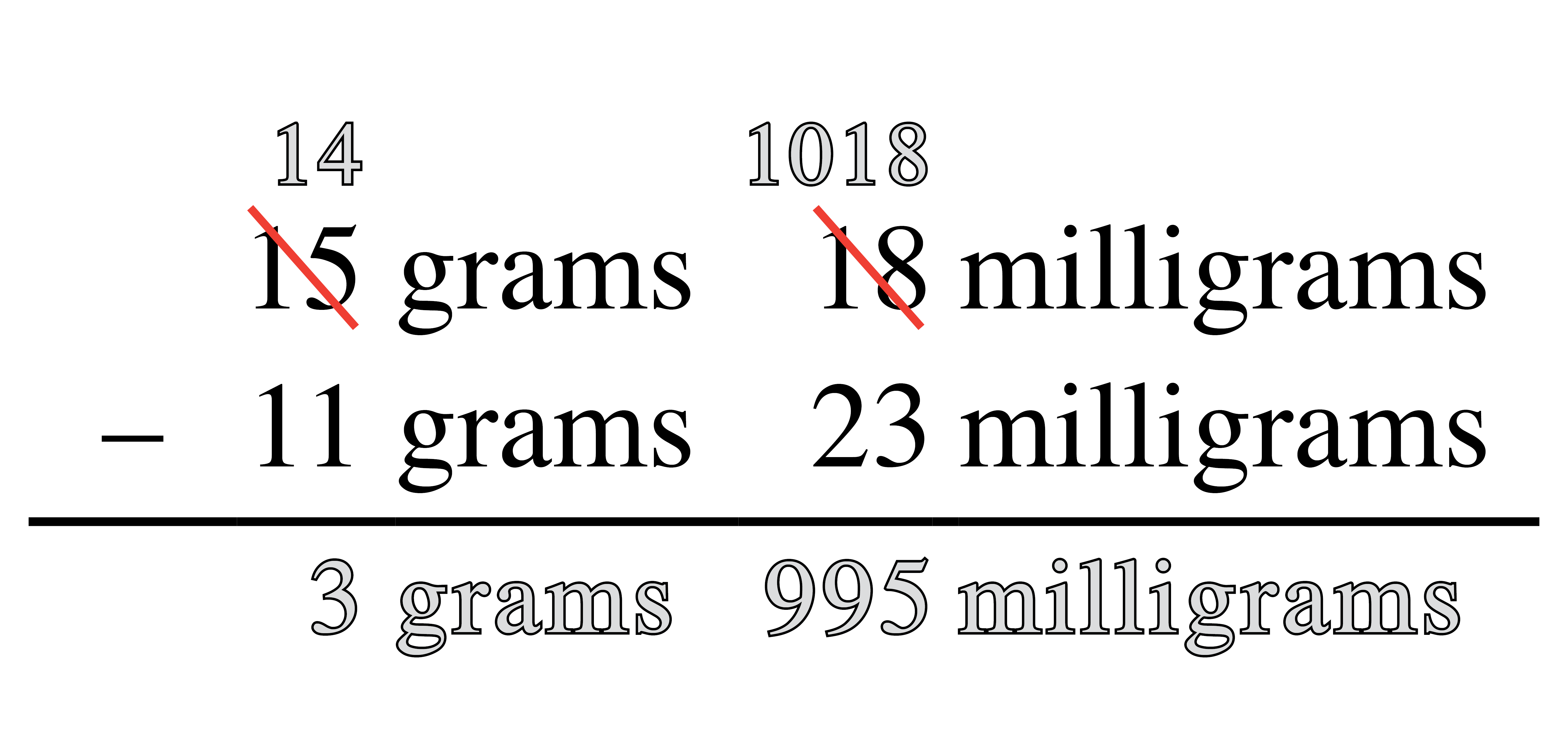
Counting
Counting is the key to unlocking addition and subtraction in early math development. At Mathnasium, our initial goal is to have a student become comfortable with counting to any number, from any number, by any number, forward and backward.
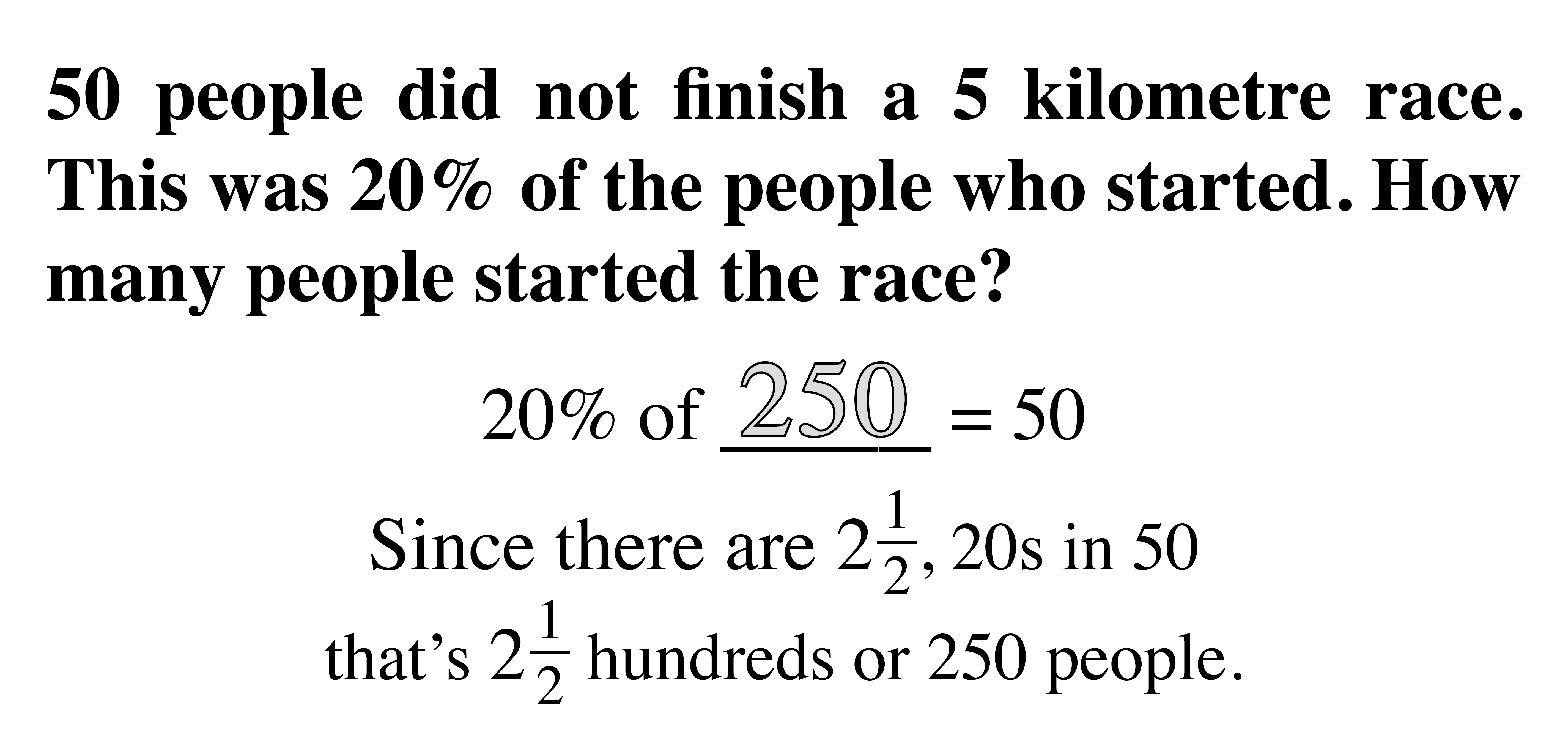
Wholes and Parts
As students begin to understand the relationship between a whole and the parts, a world of mathematical concepts and exercises can be explored. Once students have mastered these skills, they have little trouble with algebraic problem-solving.
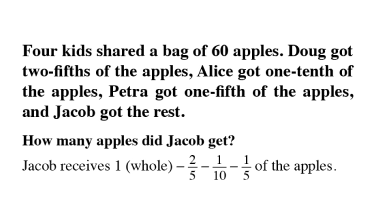
Quantity and Denomination
The quantity and denomination construct examine two aspects of numerical value. Quantity asks “how many” and denomination asks “of what.”
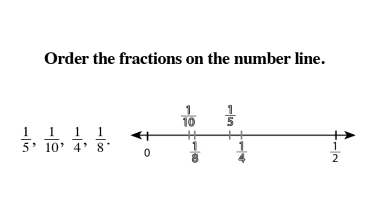
Proportional Thinking
Proportional thinking establishes a fundamental base that leads to a stronger understanding of critical concepts like ratios, direct and indirect variation, and algebraic reasoning.
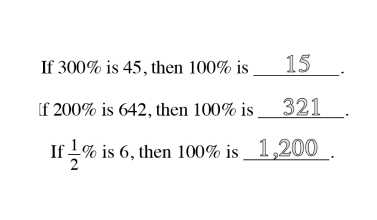
The Law of SAMEness
The Law of SAMEness is a concept students naturally apply in their reasoning without being aware of it. For example, quantities of apples and bananas cannot be added together unless first being changed so that they have the same name, which is fruit.
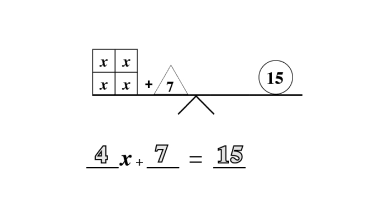
Counting
Counting is the key to unlocking addition and subtraction in early math development. At Mathnasium, our initial goal is to have a student become comfortable with counting to any number, from any number, by any number, forward and backward.
Wholes and Parts
As students begin to understand the relationship between a whole and the parts, a world of mathematical concepts and exercises can be explored. Once students have mastered these skills, they have little trouble with algebraic problem-solving.
Quantity and Denomination
The quantity and denomination construct examine two aspects of numerical value. Quantity asks “how many” and denomination asks “of what.”
Proportional Thinking
Proportional thinking establishes a fundamental base that leads to a stronger understanding of critical concepts like ratios, direct and indirect variation, and algebraic reasoning.
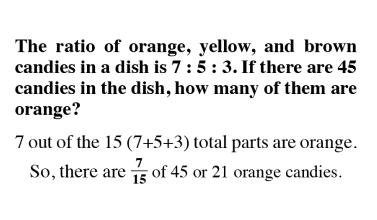
The Law of SAMEness
The Law of SAMEness is a concept students naturally apply in their reasoning without being aware of it. For example, quantities of apples and bananas cannot be added together unless first being changed so that they have the same name, which is fruit.
We use a combination of mental, verbal, visual, tactile, and written techniques to build math knowledge level by level, so they understand it, master it, and enjoy it.
The results are transformative - families will see measurable changes in attitude, confidence, and school progress.
See Our Resultsof parents report an improvement in their child’s math skills and understanding.
of parents report improved attitude toward math after attending Mathnasium.
of students saw an improvement in their school grades.
I never thought I could do math. Now I know better.
Ariella, grade 5
My kids love mathnasium Nanaimo. They learn math in a fun environment. And the staff are friendly!
I couldn't be more pleased with our experience at Mathnasium. From first meeting Ganga & Ramesh, to now, it's ...
We have over 1,000 centres in North America. Get started now.
It’s as easy as:
